S. N. Bose
Lived 1894 – 1974.
S. N. Bose founded quantum statistics in 1924 when he discovered a new way to derive Planck’s radiation law.
Bose’s method was based on the argument that one photon of light is not distinguishable from another of the same color, which meant that a new way of counting particles was needed – Bose’s statistics.
Albert Einstein extended Bose’s argument to a wider range of phenomena. Nowadays, any particle that behaves in accordance with Bose’s statistics is classed as a boson, named in honor of Bose.
Beginnings
Satyendra Nath Bose was born into a middle class family on January 1, 1894 in British India’s capital city, Calcutta, Bengal Presidency. Today the city is known as Kolkata, located in the Indian state of West Bengal.
Satyendra’s father was Surendranath Bose, an accountant in the East Indian Railway Company. Surendranath had a great interest in mathematics & science and in 1903 founded a small pharmaceutical and chemical company. Satyendra’s mother was Amodini Devi, a lawyer’s daughter. Satyendra was the couple’s eldest child and their only son; in the years following Satyendra’s birth, his parents had six daughters.
At age five, Satyendra enrolled at his local elementary school. Later, after his family moved to Calcutta’s Goabagan neighborhood, he became a pupil at the New Indian School.
Encouraging an Outstanding Pupil
His father encouraged Satyendra’s mathematical skills. Each morning, before leaving for work, he would write arithmetic problems on the floor for his son to solve. Satyendra always solved these before his father returned home.
In 1907, at age 13, Satyendra began high school at the illustrious Hindu School. He was quickly recognized as an outstanding pupil, especially in mathematics and the sciences. His mathematics teacher believed he had the potential to become a great mathematician, the equal of Pierre-Simon Laplace.
Bachelor’s and Master’s Degrees
By 1909, age 15, Satyendra Bose had completed high school. He began a bachelor of science degree at Calcutta’s Presidency College, which is located next to the Hindu School. He majored in Applied Mathematics, and again he proved to be an outstanding student, graduating in 1913 at the top of his class, with first class honors.
Bose decided he wanted to remain in academia. He enrolled for a master’s degree in Applied Mathematics at the University of Calcutta. In 1915, age 21, he graduated at the top of his class. He also learned enough scientific German and French to read works published in these languages.
Becoming an Academic
Any modern scholar at this stage in their career would enroll for a Ph.D. degree; this would be a straightforward process. However, it was less straightforward for Bose. For a start, World War 1 had begun in 1914, and European scientific journals were now arriving in India infrequently. This was frustrating, because physics in Europe seemed to be alive with new possibilities in quantum theories, atomic theories, and relativity. Another difficulty was that Ph.D. degree programs were new to the University of Calcutta.
However, things were beginning to look up for Bose and other young Indians in Calcutta who wished to study science as postgraduates. Sir Asutosh Mookerji, mathematician, and Vice Chancellor of the University of Calcutta, had been donated a large amount of money by two Calcutta lawyers to promote advanced education for Indians. In 1914, Sir Asutosh began spending the money, establishing new professorships and research programs.
Bose and other recent graduates asked Sir Asutosh to allow them to teach postgraduate courses in mathematics and physics. Sir Asutosh said this would be possible after the graduates had carried out research work themselves. He gave them scholarships for postgraduate work beginning in 1916 and placed orders for the most useful academic journals. He gave the graduate students access to his own private library of mathematics and physics books: these were more advanced than the books publicly available at the university.
Bose and his friend Meghnad Saha, who became an eminent astrophysicist, were able to source cutting-edge textbooks from Paul Brühl, an Austrian who was teaching physics at the Bengal Engineering College. With a lot of hard work, this enabled them to upgrade their knowledge to postgraduate level in electromagnetism, relativity, spectroscopy, statistical mechanics, and thermodynamics. At the end of 1916, Bose started giving applied mathematics lectures, and in 1917 he started giving mathematical physics lectures.
In 1917, the university appointed C. V. Raman to the Palit Chair of Physics.
In 1919, Bose and Saha published English translations of Albert Einstein’s special and general relativity papers.
In 1921, Bose was appointed to the position of Reader in Physics at the University of Dhaka, which is now in Bangladesh.
S. N. Bose and Quantum Statistics
Bose’s great scientific discovery came about because of his dedication to teaching. He prepared diligently for the lectures he gave to his postgraduate students and hated it if he did not fully understand everything he taught.
One problem he faced was Planck’s radiation formula. This formula was Max Planck’s solution to the failure of classical physics to account for the range of electromagnetic radiation frequencies emitted by a hot body.

Hot metal glows, emitting electromagnetic radiation.
Max Planck founded quantum theory in 1900 when he pointed out that if energy is quantized – in other words if energy only comes in lumps of certain fixed multiples – physics, which was in serious trouble, would start working again – Plank’s approach correctly predicted the radiation frequencies emitted by hot bodies.
What is Quantization?
Think about the three times table; only numbers divisible by 3 are allowed; all other numbers are forbidden. Planck proposed the same idea for the energy carried by electromagnetic radiation, except energy did not come in multiples of 3, it came in multiples of the quantity now known as Planck’s constant. To four significant figures, Planck’s constant is 6.626 x 10-34 J s. It is a tiny quantity, but its effect on physics – the principle that energy is quantized – was dramatic.
And so the 20th century began with the first hint of classical physics’ demise and the birth of quantum physics – although nobody seemed to be aware of this at first.
In 1905, Albert Einstein took Planck’s proposal of quantized energy and applied classical Boltzmann statistics to it – these describe the behavior of large numbers of gas particles. This allowed Einstein to describe the photon – the particle of light – for the first time. Einstein’s work was brilliant, but most physicists rejected the idea of photons. They said it was well-established by experiment that light was a wave.
Of course, nobody at this stage knew about wave-particle duality, the strange fact that in the quantum-world the circumstances of the observation determine whether we see a particle or a wave. Electrons, for example, sometimes show wave properties, while at other times they show particle properties.
Almost a quarter-century after Planck’s first quantum paper, Bose was preparing to teach Planck’s radiation law to a class. He was unhappy about this, because something about the theory didn’t seem right to him.
So he replaced Boltzmann’s classical statistics with his own. Where Boltzmann’s method of counting particles said that every particle was distinguishable from every other particle, Bose said otherwise. Bose’s statistics said that particles were indistinguishable from one-another.
By doing this, he was able to derive Planck’s radiation law in a way that pleased him.
Bose did not realize just how groundbreaking his work was, but he felt it was very interesting. He sent a paper entitled Planck’s Law and the Hypothesis of Light Quanta to The Philosophical Magazine, a physics journal that had already published a number of his papers. Unfortunately, like Bose, the journal’s referees did not realize that the paper was groundbreaking. In fact, they failed to see its significance at all, and rejected it.
So on June 4, 1924, Bose sent his paper directly to Einstein, writing:
 “I have ventured to send you the accompanying article for your perusal and opinion. I am anxious to know what you think of it. You will see that I have tried to deduce the coefficient 8πν2/c3 in Planck’s Law independent of the classical electrodynamics.”
“I have ventured to send you the accompanying article for your perusal and opinion. I am anxious to know what you think of it. You will see that I have tried to deduce the coefficient 8πν2/c3 in Planck’s Law independent of the classical electrodynamics.”
S. N. BOSE
1924
Einstein instantly saw that Bose’s work was very valuable, although even he did not see its full far-reaching significance at first – this took him a few days.
Planck’s quantum law actually contained a factor from classical physics: 8πν2/c3. The mixing of classical and quantum theories in one equation had been a perpetual source of discomfort for physicists working in the field. Bose had been able to produce the 8πν2/c3 factor without using classical physics. Instead the factor arose naturally from the argument that photons with equal energy were indistinguishable from one another.
Einstein translated Bose’s work into German and arranged for it to be published in the journal Zeitschrift für Physik. Einstein replied to Bose on July 24, saying that Bose’s work was:
“… an important step forward and I liked it very much.”
The Judgment of History
Bose’s paper is now recognized as one of the most important theoretical papers in the founding of quantum theory. In fact, Bose had founded an entire new field: quantum statistics.
The Effect of Bose’s Statistics
The statistics Bose introduced can seem like science fiction. In the quantum world, however, these statistics are real enough. Imagine if Bose’s statistics applied to the world we see with the naked eye.
Consider the situation when you simultaneously toss two distinguishable unbiased coins. You can write the following outcomes, all of which have the same chance of happening.
Possible Outcomes: (Two Heads) or (Two Tails) or (Head & Tail) or (Tail & Head)
The chance of getting, say, two heads is 1⁄4.
BUT, if you found it impossible to tell one coin from the other, then the coins become indistinguishable. Then you find:
Possible Outcomes: (Two Heads) or (Two Tails) or (One of Each)
The chance of getting, say, two heads is now 1⁄3.
In the world of Bose statistics, the likelihood of events happening is different from our everyday expectations.
The Bose–Einstein Condensate – A New Phase of Matter
The more Einstein thought about Bose’s paper, the more he was impressed by the possibilities it offered. He decided to apply Bose’s quantum statistics to a gas made of atoms to see what effects might be expected.
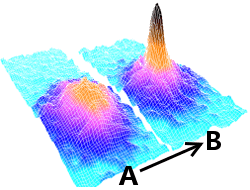
The first Bose-Einstein condensate. At time A, atoms are beginning to accumulate closely. At time B, the very dark peak indicates a region of high density; here about 2,000 atoms have merged to form a very dense ‘super-atom.’ Image from NIST.
In a BEC each particle in a collection of particles exists in the same, identical quantum state – the particles are indistinguishable from one another.
In took over 70 years from Einstein’s prediction of BECs for a pure single entity to be seen.
This happened in 1995 when a gas of rubidium atoms was cooled to within a fraction of absolute zero: 1.7 × 10-7 kelvins, merging about 2,000 individual atoms into the same entity, a single super-atom, which held together for less than 20 seconds.
Atomic Behavior During Formation of a Bose-Einstein Condensate
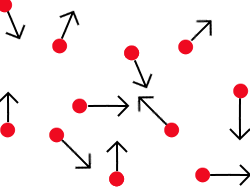
At room temperature the atoms in a gas behave like particles.

At low temperatures the wave nature of atoms increases and the atoms move closer.

As the temperature gets closer to absolute zero the waves begin to merge to form a single wave – a super-atom.

Within a millionth of a degree of absolute zero, a Bose-Einstein condensate forms – all the individual atoms have merged.
Superfluid Helium
In the quantum world there is no friction; if there were, atoms would eventually stop moving. It is only on a larger scale that friction appears.
Although not a pure BEC, superfluid helium-4 is a more familiar example of the effects of BECs than a gas of rubidium atoms.
When helium-4 is cooled to within 2 degrees of absolute zero, it behaves like a BEC. Its atoms share the same quantum mechanical state, and its quantum mechanical properties become apparent to the naked eye. At or below 2.17 kelvins, Helium-4 flows without friction.
The Boson
In December 1946, Paul Dirac coined two new words to cover the two groups of particles that exist in the subatomic world: the boson, named in honor of S. N. Bose; and the fermion, named in honor of Enrico Fermi.
Any particle that obeys Bose’s statistics is a boson, while any particle that obeys Fermi-Dirac statistics is a fermion.
Some Personal Details and the End
In 1914, age 20, Bose married Ushabala Ghosh, a wealthy physician’s daughter, who was 11 years old. The marriage had been arranged by Bose’s mother. Bose would rather have married later, but went along with his mother’s wishes. He refused to accept a dowry. The couple had nine children, seven of whom survived to be adults – two sons and five daughters.
Bose was a keen popularizer of science. In part, this was simply a love of science. Also however, he was a strong supporter of Indian independence and he believed one of the best ways to secure a prosperous future for an independent India was to have a well-educated, enlightened population.
One way he popularized science was writing and speaking about it in Bengali to ensure it was delivered to a wider audience. He ran evening classes for the children of workers.
Bose never got a Ph.D degree. He could easily have been awarded one for his invention of quantum statistics, but he was a very modest man; he was content to have made the discovery; he did not need the title ‘doctor.’
After his paper was published, he spent two years in Europe working in France and Germany. He returned to Dhaka where he became head of physics in 1927, age 33. He later wrote:
On my return to India, I wrote some papers. I did something on statistics and then again on relativity theory, a sort of mixture, a medley. They were not so important… I was like a comet, a comet which came once and never returned.
He joined the ranks of scientists who have made tremendous contributions to science, such as Willard Gibbs, Oswald Avery, Lise Meitner, and Fred Hoyle, who were never awarded a Nobel Prize.
In 1945, Bose returned to the University of Calcutta and taught there until 1956. In 1959, at age 65, he was given the honorary title of India’s National Professor. Later, as professor emeritus, he carried out nuclear physics research.
Outside of science, Bose loved poetry, music, chess, and cats.
Satyendra Nath Bose died in Calcutta, India on February 4, 1974, age 80, of bronchial pneumonia.

No comments:
Post a Comment